Fearless Symmetry
By Daniel S. Silver
A brief review of Creating Symmetry: The Artful Mathematics of Wallpaper Patterns, by Frank A. Farris
A brief review of Creating Symmetry: The Artful Mathematics of Wallpaper Patterns, by Frank A. Farris

DOI: 10.1511/2015.116.360
CREATING SYMMETRY: The Artful Mathematics of Wallpaper Patterns. Frank A. Farris. xvi + 232 pp. Princeton University Press, 2015. $35.00.
As a rule, mathematicians have an easier time with music than with art. Perhaps it is because music, like mathematics, is inherently abstract. Or perhaps it is because cultural cues are less essential to appreciating a sonata than a portrait. Whatever the reason, it seems likely that attendees of a mathematics conference could more easily cobble together a string quartet than a modest gallery show.
The imbalance wasn’t always so. During the Renaissance, painters and mathematicians recognized each other as fellow seekers of truth. The German master Albrecht Dürer, for example, devoted years to the study of geometry. Only with geometry, Dürer believed, could errors be avoided. But artists grew tired of measured proportion and mathematical perspective, with their hard rules and unforgiving nature. As Romanticism flourished in the 19th century, the marriage between mathematicians and artists became strained. They had grown apart.
Separated but not divorced, they at least continued speaking to each other. Plaster models of exotic surfaces by brilliant mathematicians such as Felix Klein and Eduard Kummer inspired revolutionary visual artists such as Man Ray and Henry Moore. The graphic artist M. C. Escher welcomed advice from mathematicians as he explored his imaginary landscapes of tessellations and impossible perspectives. But nothing since the Renaissance has done more to heal the rift than the personal computer. Its powerful graphics capability is a tool for artists as well as mathematicians. Algorithmically generated forms have replaced lines and curves. Today a mathematician without any formal art training can produce spectacular images that are unachievable with traditional tools. Our appreciation of the complex symmetries that result reminds us that symmetry itself is fundamental to human thought—just one reason why mathematics and art were always meant for each other.
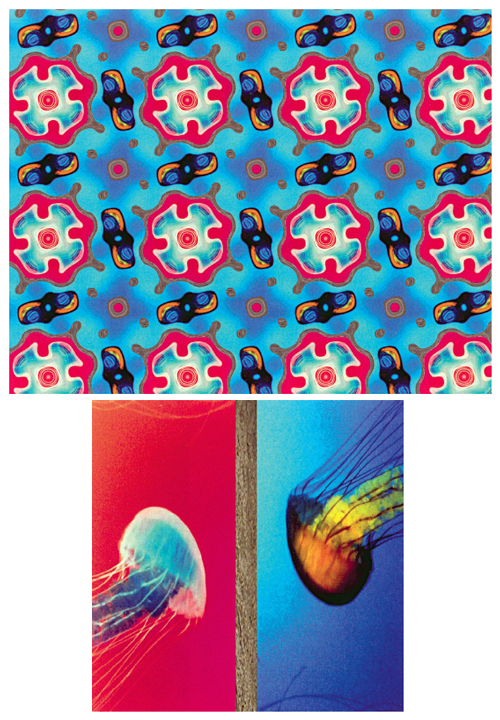
From Creating Symmetry.
Digitally savvy types who wish to create images of their own will find Creating Symmetry, by Frank A. Farris, a mathematics professor and former editor of Mathematics Magazine, to be a valuable toolbox for producing impressive two-dimensional symmetric designs. Here the painter’s palette is an ordinary color photograph, the brush a computer. A periodic function, carefully chosen, carries each point of the photograph to multiple images. The result is a replicated, finely chopped, and reassembled image, reminiscent of the sort one might see through a kaleidoscope. A plate of avocados, lettuce, and strawberries becomes a barely recognizable mélange exhibiting three-fold symmetry.
But what is symmetry exactly? And how can a function be found that will achieve a desired type of symmetry? Creating Symmetry provides answers using the language of analysis, abstract algebra, and functions of a complex variable. In its preface, the author identifies three possible classes of readers: the less experienced reader who has some understanding of calculus (Farris calls this type “the brave mathematical adventurer”), the advanced undergraduate, and the working mathematician. Much of the book is a patient review of the numerous definitions and theorems needed, presented with metaphors and analogies, friendly and reassuring. The advanced undergraduate and working mathematician will appreciate the review. The less experienced reader might be overwhelmed. Nonetheless, in explaining the crucial concept of symmetry, Farris clearly strives to bring all three groups along with him.
Mathematicians have an indirect but effective way to study designs in the plane. Instead of focusing on the design itself, they look at the collection of all rigid plane motions—compositions of rotations, mirror reflections, and sliding translations—that carry the design to itself. Such a motion is called a symmetry. Any two symmetries can be “multiplied,” performing first one and then the other, to produce another, possibly different, symmetry. Taken together, the collection of symmetries of a design is an example of a group. If the design is an equilateral triangle, for example, then rotations through 60 degrees, 120 degrees, and 360 degrees are possible. So are reflections across each of the three lines joining a vertex to the midpoint of the opposite edge. These six symmetries comprise a dihedral group.
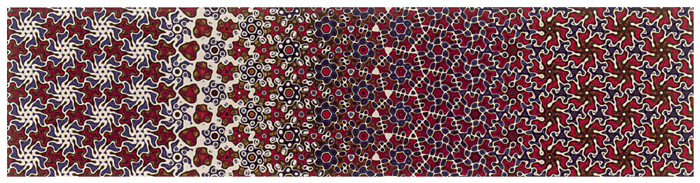
A strip design extending infinitely both to the left and the right, admitting all multiples of a translation in a single direction, is called a frieze pattern. Its group of symmetries is called a frieze group. It is known but not at all obvious that there are exactly seven such groups. If the figure covers the whole plane, admitting translations in two independent directions, then it is called a wallpaper pattern, and its group is a wallpaper group. Again, the number of such groups is known, although not obvious. There are 17. Like so many fertile subjects in mathematics, the study of symmetry arose from science—in this case, crystallography, which classifies crystals according to the patterns of their atomic structures.
Farris guides the reader through explanations and proofs of these well-known results, then gives recipes for turning a photograph into a design with any desired symmetry group. (Helpfully, an appendix gathers all the recipes presented in the book.) One of the most impressive is a method for morphing one frieze pattern into another, an effect that M. C. Escher managed without a computer in many of his miraculous prints.
Like any technical book, Creating Symmetry has its flaws. A naive reader might get the erroneous impression that the theorems here are new. It is unfortunate that no attributions are given, and very little history is presented. An experienced reader might be put off by the book’s sometimes boastful, first-person bloglike style. We learn that the author takes hikes in the Sierra Nevada Mountains and enjoys fresh fruit and vegetables, but we do not learn about any of the many mathematicians and crystallographers who are responsible for the ideas that made such a book possible—names such as William Barlow, Arthur Cayley, Evgraf Federov, Joseph Fourier, Paul Niggli, Henri Poincaré, George Pólya, and Arthur Schoenflies.
Lacking also is any mention of artistic creations by other mathematicians. Today there is an explosion of computer-generated art involving such mathematical concepts as fractal sets, cellular automata, and aperiodic tilings. Much of it is beautiful but also profound. Like the plaster models of the previous century, such art can inspire new conjectures and theorems. (A generous collection of website links where such work can be found has been compiled by Swedish mathematics teacher Bruno Kevius—see http://mathres.kevius.com/art.html.)
Despite its shortcomings, Creating Symmetry is a good source of projects for mathematics students with some computer graphics ability who wish to learn about symmetry in a truly hands-on way. It joins the endeavor of those who seek beauty through symmetry, an effort that began on the cave walls of our ancestors.
It seems fitting to close with words of mathematician and theoretical physicist Herman Weyl’s landmark 1952 essay, “Symmetry”: “Symmetry, as wide or as narrow as you may define its meaning, is one idea by which man through the ages has tried to comprehend order, beauty, and perfection.”
Daniel S. Silver is a professor in the Department of Mathematics and Statistics, University of South Alabama. Much of his research explores the relationship between knots and dynamical systems. Other active interests include the history of science and the psychology of invention.
Click "American Scientist" to access home page
American Scientist Comments and Discussion
To discuss our articles or comment on them, please share them and tag American Scientist on social media platforms. Here are links to our profiles on Twitter, Facebook, and LinkedIn.
If we re-share your post, we will moderate comments/discussion following our comments policy.