American Scientist

"All science is either physics or stamp collecting" said Lord Rutherford, who was not a stamp collector. The remark did nothing to win friends for physics among practitioners of other sciences. But Rutherford got his come-uppance: When he was summoned to Stockholm in 1908, the prize awaiting him there was not in physics but in chemistry.
A century later, surveying the state of physics and its relations with other fields, I am tempted to give Rutherford's quip an even more inflammatory reading, though he never intended it. "All science is physics" might be taken as a territorial claim, annexing other disciplines as provinces to be ruled by the laws of physics and administered by physicists. This imperial vision of the destiny of physics is not entirely without a basis in history, or at least etymology. At one time, the term physics had a very broad meaning, roughly synonymous with natural science. The 18th-century Encyclopédia of Diderot and d'Alembert listed under the rubric physique particuliere everything from astronomy and cosmology to meteorology, mineralogy, chemistry, zoology and botany (but not stamp collecting).

Browsing through recent issues of Physical Review E (a section of the main journal published by the American Physical Society), one could form an equally expansive view of the scope of 21st-century physics. Within the past year, the Phys Rev E table of contents has included titles such as "Outbreaks of Hantavirus induced by seasonality," "Large-scale structural organization of social networks," "Topology of the world trade web," "Generating neural circuits that implement probabilistic reasoning" and "Number fluctuation and the fundamental theorem of arithmetic." Evidently, the boundaries of physics are elastic enough to take in aspects of viral epidemiology, sociology, market economics, cognitive neuroscience and number theory. Are all of those fields now absorbed into the empire of physics?

The story I want to tell here is not about sleeper cells of militant physicists plotting a coup in the biology department. As a matter of fact, although physics provides the most dramatic examples, several other disciplines also have boundaries that seem to be shifting or growing porous. Intellectual migrants are wandering back and forth across many academic frontiers, generally without stopping for any formalities at the customs house. In some cases, the same paper might be classified as physics, biology, mathematics or computer science, depending more on the author's affiliation and where it was published than on the subject matter.

Departmental reshuffling and realignment goes on all the time, but the present moment seems to be one of particular ferment. Among many possible causes, I would point to the changing role of computation in the various sciences. A number of earlier upheavals in the structure of scientific disciplines have been triggered by new techniques and instruments, sometimes imported from other fields. Today, computation is the common thread in many of the areas that are having a disciplinary identity crisis. Some of these areas rely heavily on computer simulations or experiments, and others analyze large data sets accessible only with computer technology. Computer science also exerts a subtler but deeper influence when laws of nature are expressed in algorithmic form.

Social Phase Transitions

How does it happen that a sensible and sober-minded physicist strays into such dangerous neighborhoods as economics, sociology or political science? Well, one thing leads to another. The road to ruin may be long and twisting, but each step along the way is easy enough to trace.

Here's an example. Physics has a long-standing interest in the phases of matter and the transitions between those phases. This topic includes not only the familiar solid-liquid-vapor phases but also related phenomena such as the onset of magnetization in iron. One strategy for studying phase transitions is to sweep aside all the intricacy of atomic or molecular structure and build the simplest model that exhibits the behavior of interest. In the case of magnetism, the iron atom with its halo of 56 spinning electrons can be replaced by a single abstract "spin"—which is merely an arrow that points either up or down and has no other properties. The spins are arranged on a geometrical grid or lattice, a cartoon version of the crystal structure of the metal. Quantum interactions between iron atoms are modeled by a simple tendency for nearby spins to line up parallel to one another, but this orderly state can be disrupted by thermal agitation. If this rudimentary model is a success, then at some temperature most of the spins should suddenly fall into alignment, mimicking the spontaneous magnetization of a real magnet.

Having created this model to represent a specific physical system, you might now discover that the model itself is an interesting object of study. Variations suggest themselves, with different lattice geometries or rules of interaction; the variants may or may not have anything to do with magnetic materials. In some cases the behavior of the model can be worked out mathematically in full detail, but more often the only way to understand how the array of spins evolves is by computer simulation.

Now comes the next step down the path leading out of the Garden of Physics. After spending some time exploring the universe of abstract models, you may begin to notice that the lattice of spins could be given a variety of interpretations; the spins could represent many things other than magnetic moments of atoms. In particular, up and down spins might be mapped onto pro and contra opinions held by people in some social context. In this new view of the model, the interactions that were once seen as magnetic couplings now represent the tendency of people to influence (and be influenced by) their neighbors' opinions. The phase transition in which the spins all line up pointing the same way corresponds to the sudden emergence of a consensus within the population. And thus a physicist becomes a social scientist.

For another example, consider the process of percolation, where a fluid trickles through the mazelike passages of a porous medium. Can the fluid penetrate the entire region, or will it be blocked by dead-end passages? Again the essentials can be captured in a lattice model. Each link between adjacent nodes of the lattice is open to fluid flow with some fixed probability p or is blocked with probability 1-p. At low values of p, most links are blocked, and the lattice consists of many small, isolated clusters of connected nodes. As p increases, there is a threshold value where a giant connected cluster suddenly appears, allowing a fluid to infiltrate the entire lattice.

Like the lattice spin system, the percolation model has many variationsÑand many interpretations distant from the physical process that inspired it. The idea of something spreading probabilistically through a network can also model the transmission of rumors, or the progress of a forest fire or the spread of an infectious disease. Indeed, maybe the percolation model could model itself, documenting its own spread from one discipline to the next.

These are a few of the paths radiating from physics to other areas. But the landscape  of science is criss-crossed with trails going in other directions as well. A mathematician studying random graphs—structures formed when you start with a set of isolated nodes and then add links between them at random—would also discover an abrupt transition where a giant connected component spontaneously emerges. This sudden change in the structure of the graphs has all the characteristics of a phase transition, and so the mathematician wanders onto turf usually claimed by physicists.

A computer scientist could have a similar experience. The computational problem known as satisfiability concerns Boolean formulasÑlogical statements such as ((p OR q) and ((not q) OR r)), where each of the variables p, q and r has a value of either true or false. The question is: Can you find an assignment of values that makes the overall proposition true? For the example given here it's easy to answer this question by trial and error, but large formulas are challenging. In the 1980s computer scientists detected an interesting pattern: As a certain parameter measuring the complexity of the formula increases, there is a sudden transition. Below the threshold, almost all satisfiability problems are solvable, but above it almost none are. The resemblance to phase transitions is obvious, and so computer scientists found themselves doing physics, and physicists took up work on the satisfiability problem.

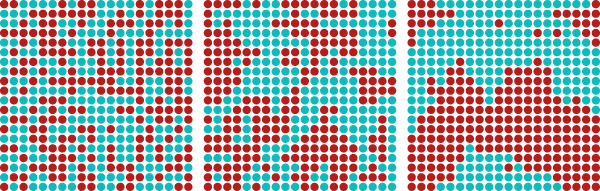
One more example from farther afield: In 1971 Thomas C. Schelling published a lattice model of racial segregation. Black and white residents, initially scattered at random over the nodes of the lattice, were assumed to prefer living among neighbors of the same race; those who were unhappy with their current surroundings could move. Schelling's most provocative finding was that it doesn't take vicious bigotry to produce a sharply segregated housing pattern; even the mildest preference for neighbors of the same race leads to a phase separation. Schelling's diagrams look very much like simulations of a lattice model of magnetic materials, but the paper makes no reference to the physics literature. (Indeed, it predates much of it.) Schelling is an economist and political scientist. 

Fishing Expeditions 

The lattice models constitute a set of problems and tools that span an impressive diversity of disciplines. But which of those disciplines is their true home? Who owns those models?

From one point of view, such a question doesn't even deserve an answer. The "intellectual property" of the pure sciences is still considered a public trust, freely available to anyone with the wit to use it. You don't have to be a licensed mathematician to write a differential equation. And unsolved problems are like fish in the sea—there for the taking by anyone who has the right bait and tackle. Nevertheless, academic communities get nervous when foreign fleets begin trawling in local waters. And no wonder. When you've been chasing the big fish all your life, it takes an uncommonly generous turn of mind to rejoice in watching someone else land it.

A scientific discipline—whether physics or mathematics or anthropology—is more than just a body of knowledge. It's also a community of people, together with the organizations and cultural traditions that bind them together—the journals they read, the meetings they attend, the jokes they tell. Such institutions resist change, and most of them are quite stable over the span of a human lifetime. But upheavals are not unknown. In retrospect these events may look exciting and rejuvenating, but some of the participants must have found them traumatic. Two historical examples worth pondering are the rise of astrophysics in the 19th century and the invention of molecular biology in the 20th.

Astronomy had its first close encounter with physics in the era of Kepler and Newton, but the consequences of that conjunction extended only to the limits of the solar system. Astronomy as applied to the stars remained the kind of science that Rutherford derided as stamp collecting. There wasn't much you could do with the stars but catalog them—give them names and note their positions, their brightness, and perhaps some hint of their color. Nothing was known of their mass and size, their composition, their age or the source of their radiant energy. The French philosopher August Comte cited the chemistry of the stars as an example of something that would remain forever unknowable.

What overturned this pessimistic assessment was an infusion of new instruments and methods, most notably spectroscopy. The discovery that narrow lines observed in stellar spectra could be matched up with corresponding lines in the spectrum of a candle flame brought the stars right into the laboratory. Almost immediately, spectroscopists were identifying chemical elements in the stars (including, in the case of helium, an element that had not yet been found on Earth). Later, subtler features of the spectra allowed inferences about temperature and pressure in stellar atmospheres, and even the measurement of stellar magnetic fields. This new style of stellar science was thoroughly multidisciplinary. There were astronomers (John Herschel) but also chemists (Robert Bunsen), physicists (Gustav Kirchhoff) and even a polymath pioneer of photography (William Henry Fox Talbot). The instigator of the whole spectroscopic revolution was an optician (Joseph von Fraunhofer). The term astrophysics, coined by the German physicist J. K. F. Zölner, must have sounded odd at the outset—as sociophysics and econophysics do today—but it has entered the mainstream now. In most universities, the Department of Astronomy is now named Astronomy and Astrophysics.

In biology, the quest to understand the molecular basis of life also involved ideas and personnel recruited from other disciplines, and yet the story is a little different. The prominent role of physicists in this undertaking is often remarked. Of the four people most closely associated with the double-helix model of DNA—Francis Crick, Rosalind Franklin, James Watson and Maurice Wilkins—three began their careers in physics or physical chemistry. Another seminal figure was Max Delbrück, who studied quantum physics with Niels Bohr before turning to biology. At least one major technology was imported from physics: X-ray crystallography became a tool for mapping the structure of biomolecules. Although Delbrück and Crick brought no new instruments with them, perhaps they passed along a physicist's style of problem-solving. Delbrück set out to find the simplest possible biological system for investigating the mechanism of heredity—he chose the bacterial viruses called phages—much as a physicist would reduce a magnet to a lattice of spins. Still, however much molecular biology may have been influenced by the physicists who helped create it, the field remains a province of biology, not a colonial outpost of physics.

In describing events like these, the choice of a metaphor makes all the difference. When physicists turned their attention to genes and proteins, did they come as a plundering horde, descending on the defenseless villages of innocent biologists? Or were they refugees from the war-blasted landscape of physics, grateful for a new home in a more peaceable realm, and eager to earn their keep by helping with the chores? Or was it an alliance, a marriage of equals but opposites, demonstrating the benefits of hybrid vigor? It would doubtless make everyone feel better if we could adopt the last of these fables, but such symmetrical unions are rare. For one thing, some disciplines just have more to export, whereas others tend to run a trade deficit. Physics and mathematics are defined as much by their methods as by their subject matter, but in fields such as geology or entomology the tricks of the trade tend to be more specialized. 

Physics Outside Physics

Will the current round of interdepartmental incursions or cross-fertilizations create new disciplines comparable to astrophysics or molecular biology? There may well be enough intellectual content for such new departments, but as yet there are few signs of the concomitant institutional changes. I have not heard of any university creating a Department of Sociology and Sociophysics.

A year ago, an international symposium held in Poland confronted the theme of "Statistical Physics Outside Physics." In an introductory talk (published, along with the rest of the proceedings, in the journal Physica A), Dietrich Stauffer of Cologne University asks what sort of welcome physicists ought to expect when they venture into economics, sociology or biology. Stauffer himself has done distinguished work in all three fields, and so the answers come from direct personal experience. And yet the question itself seems to me premature. If the work that physicists do "outside physics" is still labeled as physics—and in particular if it is still published in physics journals—then physicists may get no welcome at all. Not all sociologists, economists and biologists are readers of Physical Review E or Physica A.

The conference proceedings also include a paper by a sociologist, Barbara Pabjan of Wroclaw University, that is not exactly a warm embrace of the visiting physicists. It's understandable that social scientists are testy on this point. Their field, like a company with weak quarterly earnings, has been a constant takeover target. Even the biologists once made a bid, in the "socio-biology" movement of the 1970s.

Another newly emerging subdiscipline, bio-informatics, provides an interesting contrast. The subject matter here is the quantitative analysis  of biological data, most notably billions of base pairs of DNA sequences. The field has brought together biologists with mathematicians and computer scientists, apparently to the satisfaction of both parties. The introductory talks at bioinformatics conferences tend to focus less on friction or tension between disciplines and more on cooperation and collaboration. As far as I can tell, biologists do not worry that nerdy interlopers will poach all the best results, and mathematicians do not feel they are being exploited like some sort of outsourced tech-support hotline. Problems such as identifying genes and calculating the evolutionary distance between species are perceived as being both biologically significant and mathematically engaging. 

The Higher Stamp Collecting 

Setting aside all questions of institutional context, much of the recent cross-disciplinary work—the sociophysics as well as the bioinformatics—is fascinating and fun. Personally, when I scan Phys Rev E, it is the "unconventional" articles, the ones that transgress disciplinary boundaries, that I am likely to read first. If institutional constraints discourage such coloring outside the lines, perhaps the institutions need to be reformed.

Do we need disciplines at all? The idea of organizing universities along topical or departmental lines is not one of those long-hallowed principles without which civilization would crumble. American universities in particular resisted faculty specialization until the middle of the 19th century. Specialist journals and societies  came along even later. For example, Physical Review and the American Physical Society are not much more than a century old. (Publications for stamp collectors go back further.) Realistically, though, it is probably too late to bring back professors without portfolio.

What may still be possible is to shake up the Tree of Knowledge. As an armature for classifying ideas, a tree is a rigid structure. Its definitive feature is that branches diverge but never rejoin, so that every node can have but one parent. The proliferation of portmanteau disciplines—astrophysics, biochemistry and so on—suggests that this single-parent principle is under strain. Perhaps we should replace the tree with a matrix: Given n "prime" sciences labeling the columns and rows, we'd have cubby-holes for n ² combinations. On a campus built to reflect this architecture, you could always find your department by locating the intersection of the appropriate streets. ("Meet me at the corner of Bio and Soc.")

It's no surprise that computation is a conspicuous element in many of the recent disciplinary upsets. The computer has altered the scientist's way of life even in routine affairs (controlling experiments, communicating with colleagues, writing papers). In fields like statistical mechanics the influence is deeper. Where the aim is to understand the collective behavior of vast numbers of interacting entities, computation offers a more direct mode of investigation than has ever been possible in the past. Occasionally the role of computing gets explicit acknowledgment, as in the subdiscipline called computational chemistry. But if all science becomes computational, there's no point in mentioning it. Like mathematics, computation becomes everyone's silent partner.

Computation has even rehabilitated some of Rutherford's stamp-collecting disciplines. Those who compile lists and catalogs, who survey and classify, find their work newly glamorized in the age of data mining. The human-genome project has much to do with this change in attitude. Craig Venter, one of the principals of that project, has now begun another giant list, sailing the Sargasso Sea to create a catalog of all the organisms living there. Astronomy has its own megacatalog: the Sloan Digital Sky Survey will list 100 million objects. What has made such undertakings newly fashionable is the possibility of doing more with the data once the gigabytes have been gathered up. In a sense, the database itself becomes an object of study, in much the same way that physicists study lattices rather than what the lattices model. Rutherford might still insist that all science is either physics or stamp collecting, but maybe he would confess some interest in the physics of stamp collecting.