Explosives Detection with Nuclear Quadrupole Resonance
By Joel B. Miller, Geoffrey Barrall
An emerging technology will help to uncover land mines and terrorist bombs
An emerging technology will help to uncover land mines and terrorist bombs

DOI: 10.1511/2005.51.50
Hidden bombs pose an enormous menace. Some are responsible for such well-publicized atrocities as the downing of two Russian jetliners on the same day last August. Those in the many millions of land mines that now litter the globe garner fewer headlines on any particular day but do far more damage overall, killing or injuring more than 10,000 people each year.
How can civilized society rid itself of such threats? Clearly, the answer is complicated, but one component of the solution is to devise equipment that can reliably uncover concealed explosives before they do harm.

We have been focused on that task for many years now. Specifically, we and our many government, academic and industry colleagues are trying to develop the means for detecting explosive chemicals based on a phenomenon called nuclear quadrupole resonance (NQR). This approach offers some distinct advantages over the other options available. For example, the ability of bomb-sniffing dogs and vapor detectors to sense explosives is influenced by environmental factors such as wind and ground moisture; also, these approaches can fail with an explosive that is hermetically sealed, as is the case for some types of land mines and could readily be arranged in a terrorist bomb. And one of the most high-tech tactics tried so far—sensing the nitrogen in explosives using thermal-neutron analysis—has proved to have inadequate sensitivity and specificity. Detection through NQR does not face these difficulties. To understand why not, it is helpful to review the basic physics behind this promising technique.
Nuclear quadrupole resonance has much in common with nuclear magnetic resonance (NMR), the fundamental physical process that makes magnetic resonance imaging possible. Nuclear magnetic resonance, first demonstrated in 1946, takes advantage of the fact that certain atomic nuclei possess magnetic dipole moments—that is, these nuclei act like tiny bar magnets, each with a north magnetic pole at one end and a south magnetic pole at the other. The laws of quantum mechanics dictate that when such nuclei are subjected to an externally applied magnetic field, they must align themselves along it. But the magnetic moments of these nuclei, usually depicted as arrows, are allowed two possible orientations: in the same direction as the applied magnetic field or opposite to it.
Although alignment with the applied field is favored (this being the lower-energy condition), the energy difference between the two orientations is such that thermal agitation is usually sufficient to ensure that only slightly more than half the nuclei are in the lower-energy state. The key is that the nuclei can occupy two distinct states separated by a well-defined increment in energy. (It will be well defined as long as the applied magnetic field is uniform.) In that sense, the situation is much like that of an electron in an atom, which can be in the "ground" state or in a higher-energy "excited" state.
A ground-state electron shifts to an excited state when the atom receives a dollop of electromagnetic radiation of just the right energy to put it there—that is, when it absorbs a photon of just the right frequency. Conversely, if this excited-state electron falls back to the ground state, the atom will emit a photon of the exact same frequency to carry away the difference in energy. In NMR, the energy difference between states is much less than for the electronic states of an atom, so the relevant frequencies are much lower. Rather than dealing with optical frequencies, NMR typically involves oscillations of just a few tens to hundreds of megahertz, which includes the band where broadcast FM radio stations operate.
Stephanie Freese and Barbara Aulicino
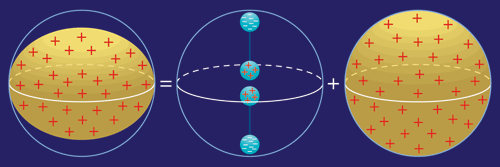
Nuclear quadrupole resonance is similar in concept, but unlike NMR it does not rely on the nuclei aligning themselves in an externally applied magnetic field. Instead, NQR exploits the fact that some nuclei possess an electric quadrupole moment, which can be thought of as arising from two back-to-back electric dipoles (positive and negative charges separated by a short distance). Why do some atomic nuclei have an electric quadrupole moment? Physicists would say because they have a spin quantum number greater than ½. A more intuitive explanation is because the positive electric charge these nuclei carry is not distributed with perfect spherical symmetry.
Consider for a moment a spherical nucleus with its positive charge distributed uniformly throughout. Now squeeze that nucleus in your mind's eye so that what was originally shaped like a basketball is flattened into a pumpkin. A pumpkin of positive charge can be thought of, to a rough approximation, as being the sum of a sphere of positive charge and two oppositely directed electric dipoles, one at the top and one at the bottom. That is, the only requirement for an electric quadrupole moment is that the nucleus be squashed (or stretched) along one axis.
When a nucleus possessing such an electric quadrupole moment is subjected to an electric field that varies from place to place, interesting things happen. The intrinsic electric quadrupole moment of the nucleus and the electric-field gradient imposed from outside together create distinct energy states. This result is analogous to the multiple energy states in NMR, where the critical ingredients were the intrinsic magnetic dipole moment of the nucleus and a magnetic field imposed from the outside.
The key difference between NMR and NQR is the definition of "outside." In NMR, the outside magnetic field arises because the experimenter has invested considerable effort in setting it up, perhaps using a superconducting electromagnet. In NQR, the required electric field (or, more precisely, the required electric-field gradient) comes for free: It reflects the local arrangement of electrons around the nucleus under study. That arrangement, in turn, depends not only on the nature of the atom but also on its chemical environment. This feature accounts for one of the chief benefits of NQR—the method is exquisitely sensitive to chemistry.
Interestingly, an early motivation for investigating NQR was the possibility that it might be useful for finding hidden explosives. Shortly after World War II, Robert Pound, one of the pioneers of NMR, became aware that people in the British army were speculating about the possibility of using this technique to detect hidden land mines. Pound was, however, skeptical that it would ever be possible to project a magnetic field of the necessary uniformity into the ground. So he decided to try NQR instead. As early as 1951, he managed to produce some promising results, but for reasons that are unclear, he did not pursue this avenue of research. A decade had to pass before others began to appreciate the potential of this idea and to study it in detail.
That later research has been carried out mostly in academic laboratories in the United States and Europe, but NQR has attracted military and commercial interest too. One of us (Miller) works at the Naval Research Laboratory, where efforts to develop NQR for the detection of explosives have been going on since 1987. The other (Barrall) is employed at a private company, Quantum Magnetics, which has been involved in similar efforts since 1993.
All of these efforts (going back as far as Pound's first tests) were predicated on the realization that, because their chemical bonds are somewhat unstable, nitrogen compounds are employed in virtually all explosives. That use has a long history. Gunpowder, for example, was first concocted some seven centuries ago from a mix of charcoal, sulfur and potassium nitrate. The 19th century saw the introduction of TNT—again another nitrogen compound: trinitrotoluene. And such modern horrors as the truck bomb Timothy McVeigh used to blow up the Alfred P. Murrah Federal Building in Oklahoma City contained the fertilizer compound ammonium nitrate.

Barbara Aulicino
Thankfully (for our purposes), the nucleus of the common isotope of nitrogen, 14N, is not spherical. It thus possesses an appreciable electric quadrupole moment and can be detected using NQR. Better yet, because the frequencies at which an NQR signal is obtained reflect the chemical environment of the nitrogen nuclei, one can distinguish dangerous explosive compounds from innocuous materials that also happen to contain nitrogen.
The basic scheme for detecting hidden explosives is fundamentally simple: One positions a loop antenna around a suspect suitcase or over a patch of mine-infested ground and applies a short pulse of radio-frequency magnetic field near the NQR frequency of interest, which is usually something less than a few megahertz. The loop antenna then serves to detect a faint return signal at the NQR frequency if the material of interest is present in the vicinity.
One complication is that the strong outgoing pulse tends to set up electrical reverberations in the antenna, just as banging on a bell with a hammer sets up mechanical vibrations that can last a long time. Although it might take only a few milliseconds for the oscillations in a typical antenna to decay to negligible levels, the return signal from some kinds of explosives lasts only a short time too. The signal one gets back from TNT, for example, has a characteristic decay time of less than one millisecond. So something must be done to ensure that the left-over oscillations from the transmitted pulse do not interfere with detection of the signal. A similar concern arises in radar equipment, where the same antenna is used to transmit powerful bursts of electromagnetic energy and to receive weak echoes from distant objects. One solution (for both radar and NQR) is to use special circuitry to dissipate the energy left in the antenna right after the transmitted pulse is finished. Another option for NQR is to use outgoing pulses that generate what are called spin echoes.
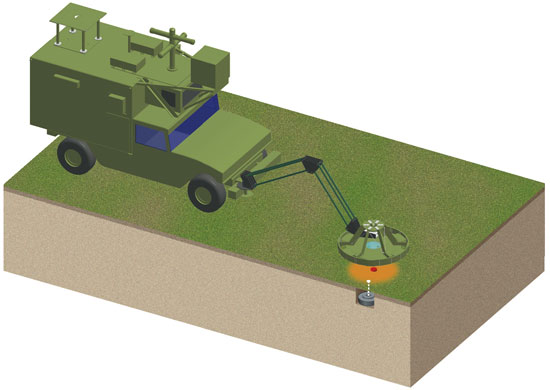
Image of truck-mounted system courtesy of Walter Freeman, Quantum Magnetics.
Spin echoes are a phenomenon unique to nuclear resonance. Their effect is to produce a measurable return from the nuclei under study after the signal has nominally died out. How in the world can that happen? The key is to understand that the reason the signal disappears in the first place is not that the individual nuclei have expended all the energy they have to give up. Rather, the overall signal is lost because the separate emanations from individual nuclei get out of synchrony. Spin echoes are induced using a specially designed sequence of pulses, ones that coax the resonating nuclei to come back into step at some later time.
A simple way to get the general idea is to imagine several runners lined up at the beginning of a road race. When the gun goes off, they all speed away from the starting line. Initially, it appears as though the runners are advancing in unison. But because some go slightly faster than others, after a short while they get out of alignment. This is analogous to what happens in nuclear-resonance experiments: The nuclei resonate at slightly different frequencies, which causes their oscillations to drift out of phase, producing little overall signal.
Now consider what would happen if the race officials instructed the runners suddenly to turn around 180 degrees and head back to where they started. At that moment, they would be at different places, but (assuming that they all kept to their established paces) eventually they would all arrive back at the starting line at the same time, the slower ones having less far to go. In nuclear resonance, a second pulse is used in essence to turn all the resonating nuclei around so that at some later moment they all get back into phase and produce a return signal that is well separated in time from the outgoing pulse.
This tactic then helps to solve the ringing-bell problem. But there is another fundamental concern in NQR: The signals are generally quite weak. Indeed they are usually comparable in magnitude to the noise that results from thermal agitation alone. So a considerable effort has to be made to extract a reliable signal from background noise.
Because thermal noise arises in a completely random fashion, one can boost an NQR signal simply by averaging the results over time or, rather, over many repeated spin echoes. The NQR signal will increase in approximate proportion to the number of spin echoes, whereas the noise will rise only with the square root of that number. More difficult is the problem presented by other forms of radio-frequency interference, which could come from, say, distant AM radio stations or from electronic equipment in the vicinity. In a controlled environment (such as within a device for inspecting baggage), one can employ suitable shielding, typically a grounded metal cage. Dealing with such radio-frequency noise is, however, a greater challenge for land-mine detection, where the space to be examined cannot be enclosed. The solution adopted at Quantum Magnetics has been to employ not one but several antennae. The additional antennae, positioned remotely from the first, are used to record the radio-frequency background at the moment the NQR measurements are taken. This noise is then digitally subtracted from the signal obtained from the main antenna.
Although this technique shows a great deal of promise as a means for finding hidden explosives, military tacticians consider NQR detectors to be too slow to be useful in clearing roads of land mines. In recognition of this concern, we are designing NQR equipment to function as a "confirmation sensor." These devices will supplement the conventional tools now being applied to the task of finding land mines: metal detectors and ground-penetrating radar, which can be quite sensitive but tend to produce many false alarms. The idea is that an NQR sensor will be used to test for the presence of explosives only at those spots identified as suspicious by these other methods, reducing the number of false alarms.
In an effort to gauge the effectiveness of NQR in this context, we and some of our colleagues arranged in 2003 to test a prototype confirmation sensor (built by Quantum Magnetics) under realistic conditions. This equipment was designed to detect antitank and antivehicle mines buried in roads. We performed these experiments at two U.S. government test sites, one situated in the desert, the other located in a temperate environment, so as to be able to gauge whether damp soil, which can interfere with conventional detection methods, posed special problems for NQR. (It didn't.)
Barbara Aulicino
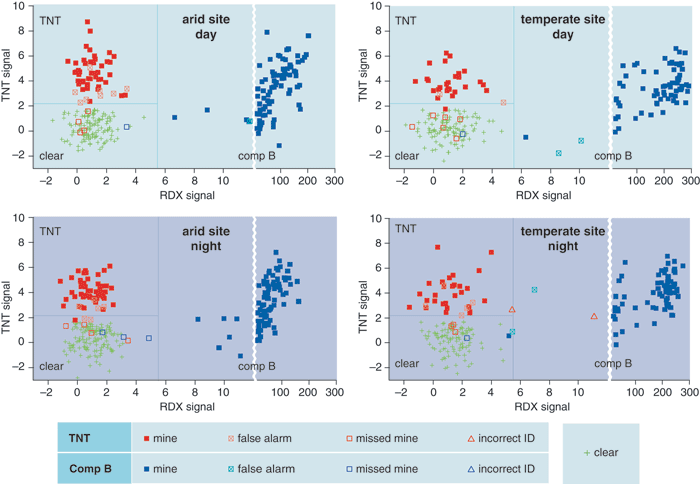
We used a variety of mines for these trials: Some contained from 5 to 8 kilograms of TNT, whereas others used anywhere from 2 to 10 kilograms of an explosive called "Comp B," which is a combination of TNT (40 percent) and the explosive compound cyclotrimethylene trinitramine, better known as Royal Demolition Explosive or RDX (60 percent). The mines were buried at realistic depths, varying from 2.5 to 12.5 centimeters (as measured from the surface of the ground to the top of the mine). These were blind tests, in the sense that the people operating the NQR equipment did not know ahead of time which of the hundreds of spots they examined held mines.
We carried out the first set of trials at the desert site, both during the day and at night. Why test day and night? Because we anticipated that radio-frequency interference would pose a bigger problem at night than during the day. (Recall how many more stations your short-wave radio picks up after the sun goes down.) Fortunately, the equipment dealt with this interference well, and the results for day and night proved to be statistically identical: The overall probability of detection was about 95 percent, and the probability of false alarm was only between 4 and 7 percent.
In the second test at the temperate site, the TNT detection probability was slightly reduced compared with what we had determined under arid conditions. But we obtained similar results at both sites for RDX. And again, the day and night tests gave nearly identical outcomes: The overall probability of detection was once more around 95 percent, and the probability of false alarms was about 5 percent.
These tests clearly showed the feasibility of detecting antitank land mines by NQR, but antipersonnel mines are a different matter. Many antipersonnel land mines contain as little as 50 grams of explosive, pushing current NQR detection sensitivity to its limits. The 2003 tests made apparent some of the practical difficulties that still limit NQR detection sensitivity. For the past few years, we and our colleagues at the Naval Research Laboratory and at Quantum Magnetics have worked (with support from the Army, the Marine Corps and the Office of Naval Research) to overcome these problems with an eye to developing rugged, portable hardware that can detect mines swiftly and reliably under harsh field conditions. We've made excellent progress in improving the sensitivity of our NQR detectors, while at the same time making them more immune to radio-frequency noise. These advances are bringing the NQR detection of antipersonnel land mines into the realm of possibility.
Of course, hidden explosives come in forms others than land mines. Fortunately, one can design NQR detector coils to search for these threats using fundamentally the same technology used to reveal land mines. Instead of passing a small coil over a large patch of ground, one typically moves a small (or perhaps not-so-small) object through a large coil. Indeed, the coil can be quite sizable. Members of the Defense Science and Technology Laboratory of the United Kingdom recently constructed an NQR system for detecting ammonium nitrate-based explosives hidden in the trunks of cars or the backs of vans. They showed that a reasonable amount of the radio-frequency field penetrates the vehicle, which is not too surprising when one remembers that portable AM radios work just fine inside most cars. They then demonstrated how a suspect vehicle could be driven into a huge detector coil and rapidly scanned for the explosives.
Barbara Aulicino
Our initial efforts in NQR for the detection of explosives were accelerated by the downing of Pan Am Flight 103 over Lockerbie, Scotland, in 1988. Soon afterward, Miller and his colleagues at the Naval Research Laboratory, with support from the FAA, began work on an NQR system capable of scanning carry-on-sized baggage for the presence of RDX-based explosives. This work showed that relatively small quantities of this explosive (but enough to be a threat to aircraft) could be detected in a reasonable time. Subsequently, the Naval Research Laboratory licensed this technology to Quantum Magnetics, which then built various prototype systems to scan larger baggage for a range of explosive materials.
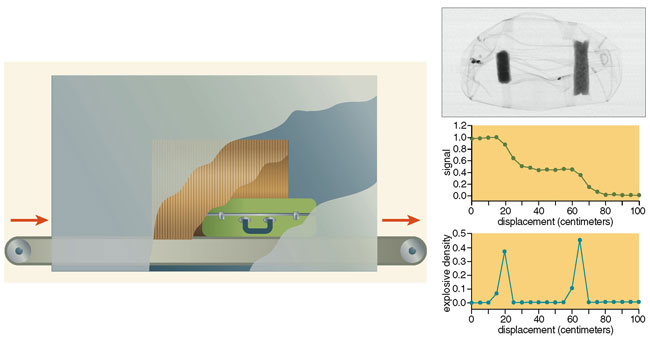
One component of NQR research at Quantum Magnetics has had the goal of not only detecting the presence of an explosive substance but also pinpointing its position within the suspect bag. It turns out that localization, at least in one dimension, is easy enough to accomplish. It requires only that many measurements be taken as the bag passes along a conveyor belt through the NQR scanner. With these observations, and knowing the physical characteristics of the coil antenna used, one can readily calculate the position of an explosive object (or objects) within the piece of luggage. Finding the position of a problematic mass in two dimensions is not difficult either: One needs only to rotate the bag 90 degrees and scan it again. Indeed, a complete three-dimensional mapping can be accomplished by rotating the bag a third time and scanning it once more. (Actually, the results can be made more accurate by using a larger number of scans, each one obtained after rotating the suspect item into a different orientation.)
Of course, running a piece of luggage through a scanner many times is bound to be tedious and time-consuming, particularly because the operator would have to take care to adjust the orientation properly during each pass. But the solution is straightforward: Run the bag though once using multiple coil antennae of different orientations. Quantum Magnetics has recently designed a system that employs two perpendicular coils oriented at 45 degrees to the direction of the conveyor belt. Although this arrangement does not allow the geometry of an explosive to be mapped in any great detail, it does provide two-dimensional localization in a single pass.
Although the ubiquity of 14N in explosives makes NQR well suited for detecting them, revealing hidden bombs is by no means the only application of this technique. Narcotics, too, frequently contain 14N, which opens the possibility of detecting smuggled drugs of abuse. We have demonstrated detection of heroin and cocaine in reasonable quantities with good sensitivity. However, the great specificity of NQR, useful in differentiating explosives and narcotics from other materials, can sometimes be a liability. In particular, the detection of illicit drugs becomes rather complicated because they exist in more than one form and because their purity varies widely, causing NQR resonance frequencies to shift and to broaden.
Although such changes are problematic for the detection of narcotics, this phenomenon suggests another potential application of NQR: for quality control in the chemical and pharmaceutical industries. Work at the Naval Research Laboratory has shown, for example, that the width of the NQR resonance lines in the explosive RDX correlates with its sensitivity to detonation, an important parameter in formulating explosives that are safe to handle.
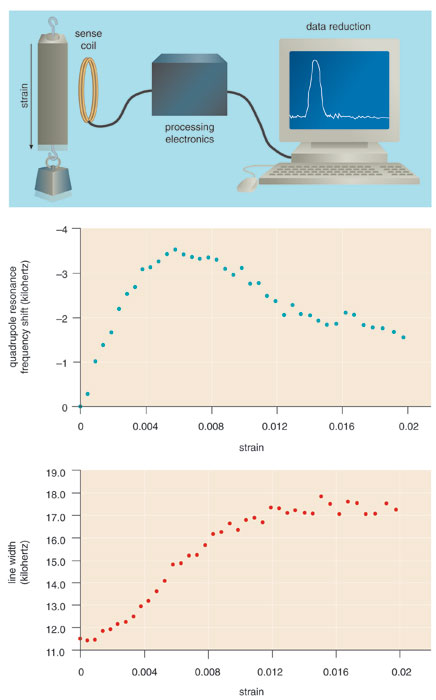
Barbara Aulicino
In many crystalline substances, defects in the orderly packing of atoms introduce strain at a microscopic scale, which in turn influences the frequencies (and frequency ranges) of the NQR resonance. Strain also can be induced by outside forces, and where and how it builds up in structural materials can have especially important consequences—namely mechanical failures. Not surprisingly, a large sub-field in engineering is devoted to the nondestructive evaluation of strain, an area in which NQR holds great promise. For example, NQR may be especially valuable for testing fiber-reinforced composite materials, which are found in everything from tennis rackets to aerospace components. These materials are not highly crystalline and usually do not contain a significant number of quadrupolar nuclei, so they would not typically provide an NQR signal.
This problem can be overcome in two ways: by embedding a small amount of a crystalline substance containing quadrupolar nuclei during manufacture of the composite material, or by later applying a coating of such a substance to the finished structure. Tests on fiberglass composites with embedded strain-sensing crystals, performed last year at Quantum Magnetics, showed that NQR indeed provides a very sensitive method of nondestructive evaluation.
The phenomenon of NQR allows, in principle, for even more ambitious applications. For example, a number of research groups, including those of Daniel J. Pusiol (National University of Córdoba in Argentina), Rainer Kimmich (Ulm University) and Bryan H. Suits (Michigan Technological University), have demonstrated the potential for NQR imaging and for the spatial localization of strain. These early efforts suggest that it may one day be possible to obtain high-resolution images showing the distribution of everything from temperature and strain state to chemical composition and purity. Given the rapidity with which MRI moved out of the laboratory and into hospitals, it seems fair to wonder: Will the benefits of NQR prove great enough to spur similarly dramatic advances?
Click "American Scientist" to access home page
American Scientist Comments and Discussion
To discuss our articles or comment on them, please share them and tag American Scientist on social media platforms. Here are links to our profiles on Twitter, Facebook, and LinkedIn.
If we re-share your post, we will moderate comments/discussion following our comments policy.