American Scientist

LEWIS CARROLL IN NUMBERLAND: His Fantastical Mathematical Logical Life. Robin Wilson. xii + 237 pp. W. W. Norton, 2008. $24.95.

Charles Lutwidge Dodgson, who wrote under the pen name Lewis Carroll, was an Oxford don, a talented mathematician, a deacon in the Church of England and a pioneer in portrait and studio photography. He is best known as the author of Alice’s Adventures in Wonderland and Through the Looking-Glass, which are, on one level, books for children. Within them, however, are philosophical discussions, sophisticated word plays, parodies, satirical comment and mathematical allusions. In Lewis Carroll in Numberland, Robin Wilson focuses on Dodgson’s personal life and particularly on his mathematical life, asking, “What mathematics did he do? How good a mathematician was he, and how influential was his work?”

In a nod to the playful nature of Dodgson’s writing style, Wilson divides his own text not into chapters but into eight “fits,” after Dodgson’s extended poem The Hunting of the Snark: An Agony in Eight Fits. Wilson’s fits follow the chronology of Dodgson’s life as they delve into his mathematical pursuits and other activities. The final two deal with puzzles, problems, paradoxes and logic.

Wilson is insightful, and his approach to his subject is scholarly and serious. The portrait of Dodgson that emerges is intriguing. The book shows him to have been an earnest and pious churchman, a dedicated teacher and popularizer of mathematics, an artistic and creative pioneer in photography, and a diffident and stammer-prone adult. Dodgson took his vows of celibacy very seriously, Wilson emphasizes; his opinion is that Dodgson would never have engaged in inappropriate behavior or done anything “untoward” in his friendships with children, whom he regarded as “the embodiment of purity.”

Dodgson’s character and his place in the world influenced every aspect of his life. Because of his shyness and insecurity, he was conservative and unadventurous in his dealings with adults. He got on better with children, especially girls, apparently because they didn’t judge him so harshly; it also helped that they weren’t anything like the boys he was forced to compete with for leadership roles when he was a pupil at Rugby School.

Dodgson was also conservative as a mathematician. He wrote pamphlets for his pupils that clarify and explain the text of Euclid’s Elements and provide exercises. When a movement sprang up advocating the use of texts other than the Elements to teach geometry, Dodgson wrote Euclid and his Modern Rivals in response, a play in four acts that shows the Elements to be superior to 13 rival texts. Wilson devotes much of his fourth fit to this controversy over alternative teaching resources. The movement for change was led by the great mathematicians James Joseph Sylvester (who attributed his childhood hatred of geometry to the study of Euclid) and Augustus De Morgan (who asked whether reliance on Euclid was more of a hindrance and a delusion than anything else). An Anti-Euclid Association was formed.

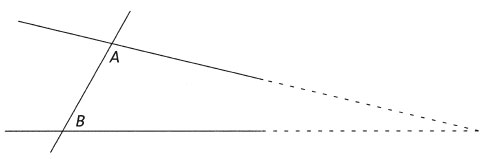
Much of the concern about the place of Euclid in mathematical thinking centered on the fifth postulate (see diagram above). An equivalent postulate states, “Given a line L and a point P not on it, there is only one line parallel to L that passes through P.” If the sort of geometry we want requires this parallel line to be unique, then Euclid’s fifth postulate is required. However, those who had questioned whether the fifth postulate was self-evident were unable to derive it from the first four.

That no such derivation is possible had been established by 1830 by Carl Friedrich Gauss, Nikolai Lobachevsky and János Bolyai, who all constructed non-Euclidean geometries in which the first four postulates hold but not the fifth. The remarkable discovery that Euclidean and non-Euclidean geometries are equally valid changed the philosophical view of mathematics, but it largely passed Dodgson by, because he deemed non-Euclidean geometries irrelevant to our physical world. Donald Coxeter, in his introduction to the Dover Edition of Euclid and his Modern Rivals, speculates on what the consequences might have been if English mathematicians Arthur Cayley and William Kingdon Clifford, who understood these new ideas, had met Dodgson and explained them to him.

Dodgson’s belief in the immutable truth of Playfair’s axiom (as the accepted modern formulation of the fifth postulate is known) led him to write A New Theory of Parallels, in which he replaced the fifth postulate with an axiom he believed to be self-evident, basing his proof on the diagram shown.

After the popular success of Alice’s Adventures in Wonderland, Queen Victoria is said to have asked to be sent a copy of Lewis Carroll’s next book. As Wilson recounts, this is how the Queen came to receive a copy of An Elementary Treatise on Determinants. She should have felt honored, because it is arguably the best example of Dodgson’s mathematical writing. It contains what is believed to be the first published proof of what is usually referred to as the Kronecker–Capelli theorem, which gives the conditions under which a system of linear equations has a solution.

Dodgson’s contributions to mathematics include several studies of systems of voting and political representation. His popularity as an author gave him an audience for his expositions on mathematics and for a series of puzzles that he produced (he awarded levels of honors to successful solvers). In Pillow-Problems Thought Out During Sleepless Nights, he presented a series of questions that were supposed to be answerable without paper and pencil—with one’s head on the pillow. Although many of these look silly from a modern viewpoint, some are gems. Pillow-Problems is a window into the mathematics of Dodgson’s day and into his character. This is well illustrated by his explanation that he intended the book to be a remedy against thinking impious thoughts before going to sleep:

There are sceptical thoughts, which seem for the moment to uproot the firmest faith; there are blasphemous thoughts, which dart unbidden into the most reverent souls; there are unholy thoughts, which torture, with their hateful presence, the fancy that would fain be pure.

I learned a great deal from this book and was able to satisfy my curiosity on two points. I had always wondered what Dodgson’s young friends thought about him. It now appears that, to children, he was not the boring pedant that some of his Oxford students thought him to be, but a humorous and kindly storyteller. I had also wondered what the Reverend Henry Liddell, the Dean of Christ Church College at the University of Oxford (and the father of Dodgson’s young friend Alice), thought of Dodgson, guessing (incorrectly) that Liddell regarded him as an inferior. Actually, Liddell’s treatment of Dodgson was always that of a good employer; he knew the man to be a talented member of his college and supported him generously.

Dodgson led a very busy mathematical life, and this book has plenty of material for a general reader to explore. I would have liked to see more critical commentary on Dodgson’s work in the context of what was happening in mathematics in the rest of the world. But this is a minor quibble. Dodgson’s remarkable intellect shines through the book. His contributions were brilliant even when they were flawed. This very nice book gives new insight into the life, the character, the stories and above all the mathematics of its remarkable subject.

John C. Butcher is Honorary Research Professor in the Department of Mathematics at the University of Auckland in New Zealand. He is the author of Numerical Methods for Ordinary Differential Equations, which is now in its second edition (Wiley, 2008).